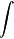The Magnetic Field part 1

As previously stated, magnetic field are the fundamental mechanism by which energy is converted from one form to another in motors, generators, and transformers. Four basic principles described how magnetic fields are used in  these devices;

1) A current-carrying wire produces a magnetic field in the area around it.

2) A time-changing magnetic field induces a voltage in a coil of wire if it passes through that coil. (this is the basis of transformer action.)

3) A current-carrying wire in the presence of a magnetic field has a force induced on it. (this is the basis of a motor action)

4) A moving wire in the presence of a magnetic field has voltage induced in it. (this is the basis of generator action.)

This section describes and elabrates on the production of a magnetic field by a current-carrying wire.

Production of a Magnetic Field

The basic law governing the production of a magnetic field by a current is Ampere's law:

  H.dl = Inet

Where H is the magnetic field intensity produced by the current Inet, and dl is a differential element of lenght along the path of integration. In SI unit, I is measured by amperes and H is measured in ampere-turns per meter.

Let's imagine a ferromagnetic core with the shape of a square and middle side is empty. Then, one of the leg is wrapped with N turn and current passing through it. This ferromagnetic core has A cross-sectional area.

So, there will be an equation;

H.lc = N.i

lc = mean path lenght of the core

i = is current passing through the wrapped coil

Then, H become ;

H = N.i / lc

The magnetic field intensity H is in a sense a measure of the "effort" that a current is putting into the establishment of a magnetic field. The strenght of the magnetic field flux produced in the core also depends on the material of the core. The relationship between the magnetic field intensity H and the resulting magnetic flux density B produced within a material is given by

B = µ.H

where

H = magnetic field intensity 

µ =  magnetic permeability of material

B = resulting magnetic flux density produced

The actual magnetic flux density produced in a piece of material is thus given by a product of two terms:

H, representing the effort exerted by the current to establish a magnetic field 

µ, representing the relative ease of establishing a magnetic field given material

The units of magnetic field intensity are ampere-turns per meter, the units of permeability are henrys per meter, and the units of the resulting flux density are webers per square meter, known as Tesla (T).

The permeability of free space is called µ0 , and its value is 

µ0 = 4π×10−7 H·m−1

The permeability of any other material compared to the permeability of free space is called its relative permeability :

μr = μ / μ0

Will be continued...

Machinary Principles

Rotational Motion, Newton's Law, And Power Relationships

Almost all electric machines rotate  about an axis, called the shaft of the machine. Because of the rotational nature of machinery, it is important to have a basic understanding of rotational motion. In this part, we will talk about a brief review of the concepts of distance, velocity, acceleration, Newton's law, and power as they apply to rotating machinery.

In general, a three-dimensional vector is required to completely describe the rotation of an object in space. However, machines normally turn on a fixed shaft, so its motion is restircted to one angular dimension. In the motion principle, direction of rotation can be described as either clockwise (CW) or counterclockwise (CCW). Counterclockwise angle of rotation is assumed to be positive, and a clockwise angle of rotation is assumed to be negative.

Angular Position θ 

The angular position θ of an object is the angle at which it is oriented, measured from some arbitrary reference point. Usually, it is measured in radians or degrees. 

Angular Velocity ω

Angular velocity or speed is the rate of change in angular position with respect to time. It is positive if the rotation is in a counterclockwise direction. Angular velocity is the rotational analog of the concept of velocity on a line. 

ω = dθ /dt

If the units of angular position are radians, then angular velocity is measured in radian per second.

In dealing with ordinary electric machines, engineers often use units other than radians per second to describe shaft speed. Frequently, the speed is given in revolutions per second or revolutions per minute. Because speed is such an important quantity in the study of machines, it is customary to use different symbols for speed when it is expressed in different units. This symbols are used to describe angular velocity : 

ωm  = angular velocity expressed in radians per second

fm      = angular velocity expressed in revolutions per second

nm     = angular velocity expressed in revolutions per minute

nm = 60fm 

fm  = ωm  / 2π

Angular Acceleration

Angular acceleration is the rate of change in angular velocity with respect to time. It is assumed positive if the angular velocity is increasing in an algebraic sense. Angular acceleration is defined by 

α = d ω / dt

If the units of angular velocity are radians per second, then angular acceleration is measured in radians per second squared.

Torque τ

In linear motion, a force applied to an object causes its velocity to change. In the absence of a net force on the object, its velocity is constant. The greater the force applied to the object, the more rapidly its velocity changes.

There exists a similar concept for rotation. When an object is rotating, its angular velocity is constant unless torque is present on it. The greater the torque on the object, the more rapidly the angular velocity of the object changes.

The torque on an object is defined as the product of the force applied to the object and the smallest distance between the line of action of the force and the object's axis of rotation. If r is a vector pointing from the axis of rotation to the point of application of the force, and if  F is the applied force, then the torque can be described as

τ=(Froce applied)(Perpendicular distance)

=(F)x(r)

=F.r.sinθ

where θ is the angle between the vector r and the vector F. The direction of the torque is clockwise if it would tend to cause a clockwise rotation and counterclockwise if it would tend to cause a counterclockwise rotation.

Newton's Law of Rotation

Simply, force applied on an object and its resulting acceleration.

F =  net force applied on an object

m = mass of the object

a =  resulting acceleration

F = m x a

A similar equation describes the relationship between the torque applied to an object and its resulting angular acceleration. This is called Newton's law of rotation ; 

τ = is the net applied torque in newton-meters or pound-feet

I  = serves the same purpose as an object's mass in linear motion. (moment of inertia)

α = is the resulting acceleration in radians per second squared

τ = I α

Work

For linear motion, work is defined as the application of a force through a distance. In equation form,

W =F.dr

For rotational motion, work is the application of a torque through an angle. Here the equation for work is

W =τ.dθ 

and if the torque is constant 

W = τθ 

Power P

Power is the rate of doing work, or the increase in work per unit time. The equation for power is

P = dW / dt

it is usually measured in joules per second (watts), but also can be measured in foot-pounds per second or in horsepower.

By this definition, and assuming that force is constant and collinear with the direction of motion, power is given by

P = dW / dt

     =d(F.r) / dt

        = F ( dr / dt )

= F.v   

Similarly, assuming constant torque, power in rotational motion is given by

P = dW / dt

    =d(τθ) / dt

    =τ(dθ / dt)

P =τω

This last power equation is very important in the study of electric machinery, because it can describe the mechanical power on the shaft of a motor or generator. This equation is the correct relationship among power, torque, and speed if power is measured in watts, torque in newton-meters, and speed in radians per second. If other units are used to measure any of the above quantities, then a constant must be introduced into the equation for unit conversion factors. It is still common in U.S. engineering practice to measure torque in pound-feet, speed in revolutions per minute, and power in either watts or horsepower. If the appropriate conversion factors are included in each term, then equation becomes

P (watts) = torque (Ib-ft) n (r / min) /  7.04

P (horsepower) = torque (Ib-ft) n (r / min) / 5252

where torque is measured in poun-feet and speed is measured in revolution per minute.

The Per-Unit System

           The SI units just described enable us to specify the magnitude of any quantity. Thus mass is expressed in kilograms, power in watts, and electric potential in volts. However, we can often get a better idea of the size of something by comparing it to the size of something similar. In effect, we can create our own unit and specify the size of similar quantities compared to this arbitrary unit. This concept gives rise to the per-unit method of expressing the magnitude of a quantity.

               For example, suppose the average weight of adults in Istanbul is 130 Ib. Using this arbitrary weight as a base, we can compare the weight of any individual in terms of this base weight. Thus a person weighing 160 Ib would have a per-unit weight of 160 Ib / 130 Ib = 1.23. Another person weighing 115 Ib / 130 Ib = 0.88.

             The per-unit system of measurement has the advantage of giving the size of a quantity in terms of a particularly convenient unit, called the per-unit base of the system. Thus in the reference to our previous example, if a wrestler has a per-unit weight of 2.0 we know his weight is far above average. Also, his real weight is 2.0 x 130 Ib = 260 Ib.

       Note that whenever per-unit values are given, they are always pure numbers. So, it would be nonsensical to express that the wrestler weighs 2.0 Ib. His weight is 1.7 per-unit, where the selected base unit is 130 Ib.

Per-Unit System with one base

           If we select the size of only one quantity as our measuring stick, the per-unit system is said to have a single base. The  may be a power, a voltage, a current, or a velocity. For example, suppose that three motors have power ratings of 25 hp, 40 hp, and 150 hp. Let us select an arbitrary base power PB of 50 hp. The corresponding per-unit ratings are then 25 hp / 50 hp = 0.5, 40 hp / 50 hp = 0.8 and 150 hp / 50 hp = 3. Thus, in this per-unit world where the base is 50 hp, the three motors have power ratings of 0.5, 0.8 and 3 pu, respectively.

3500 / 1500 = 2.33

With the using of per-unit system we can easly solve so many problems which are complex.

           We could equally well have selected a base power of 15 hp. In this case the respective per-unit rating would be 25 hp / 15 hp = 1.67, 40 hp / 15 hp = 2.67, and 150 hp / 15 hp = 10. 

          It is therefore important to know the magnitude of the base of the per-unit system. If we do not know its value, the actual values of the quantities we are dealing with cannot be calculated.

          For example, in a circuit there are R1= 3500 Ω, R2= 450 Ω, XL= 4800 Ω, and XC= 3000 Ω

R1(pu) = 3500 / 1500 = 2.33

R2(pu) = 450 / 1500 = 0.30

XL(pu) = 4800 / 1500 = 3.2

XC(pu) = 3000 / 1500 = 2 

           With the using of per-unit system we can easly solve so many problems which are complex.

          

 

How Hydroelectric Power Plant Works

       Hydropower is considered to be a renewable energy source because it uses the continuous flow of water without using up the water resource. It is also nonpolluting, since it does not rely on burning fossil fuels. Hydropower is currently the leading renewable energy source in the United States. In 2009, it accounted for about 63 percent of all other renewable energy sources, such as wind, solar, and biomass. Reclamation is the nation’s second largest producer of hydroelectric power, with 58 hydroelectric power plants and 194 generating units in operation and an installed capacity of 14,693 MW. Almost all suitable sites for dams have already been developed, so there is not much scope for further growth in water power. However, there are numerous areas where research can lead to increases in the efficiency and reliability of hydroelectric plants and decreases in maintenance costs. Presently, wind and solar energy are growing at a rapid rate, and in a near future they will be the major sources of renewable energy for production of electric power.

The hydroelectric power plants usually require a dam to store water, a pen-stock for delivering the falling water, electric generators, a valve house which contains the main sluice valves, automatic isolating valves, and related control equipments. Also, a surge tank is located just before the valve house to protect the penstock from a pressure surge, called water hammer, in case the turbine gates are suddenly closed. In addition to electric energy production, most dams in the United States are built for other uses, including recreation, irrigation, flood control, and public water supply. A schematic diagram of a hydroelectric power plant is shown in Figure 1.6.

Figure 1.6

converts mechanical energy into electrical energy. After passing through the turbine, the water reenters the river on the downstream side of the dam. The most significant operating characteristics of hydropower plants are rapid start-up and loading, long life, and low operating and maintenance costs. Hydraulic turbines, particularly those operating with a low pressure, operate at low speed. Their generators are usually salient-type rotor with many poles. To maintain the generator voltage frequency constant, the turbine must spin the generator at a constant speed given by

                                                                        n = 120f / p

where f is the generated voltage frequency and p is the number of poles of the generator.

The potential energy of the water in the reservoir is proportional to the mass of water and the difference in height between the water impoundment and the water outflow. This height difference is called the head or effective head. That is, P.E = mgh. The mass of water is its volume times its density. Therefore, P.E =

volume x p gh and the available hydro power becomes

                                                                   

                                                                     Pw = P.E / t

Pw = volume x pgh / t

Pw = qpgh W

p = density of water in kg / m3 (1000 kg/m3)

q = rate of flow of water in m3 / s

h = effective head of water in m

g = acceleration of gravity (9,81 m/s2)

With the knowledge of the above g and p values,

P = 9,81.q.h

Let's get n is an overall efficiency of the hydropower plant, the electrical power output in kW is

Po = 9,81.q.h.n

where n is

n = np.nt.ng

np is penstock efficiency

nt is turbine efficiency

ng is generator efficiency

Turbines will be explained other edition of Power Plant

Units in Life and Electricity (part 1)

        Units play an important role in our daily lives. In effect, everything we see and feel and everything we buy and sell is measured and compared by means of units. Some of these units have become so familiar that we often take them for granted, seldom stopping to think how they started, or why they were given the sizes they have.

        Centuries ago the foot was defined as the length of 36 barleycorns strung end to end, and the yard was the distance from the tip of King Edgar's nose to end of his outstretched hand.

        Since then we have come a long way in defining our units of measure more precisely. Most    units   are now based upon the physical laws of nature, which are both invariable and reproducible. Thus the meter and yard are measured in terms of the speed of light, and time by the duration of atomic vibrations. This improvement in our standards of measure has gone hand in hand with the advances in technology, and the one could not have been achieved without the other.

Getting used to SI

        The official introduction of the International System of Untis, and its adoption by most countries of the world, did not, however, eliminate the systems that were previously employed. Just like well-established habits, units become a part of ourselves, which we can not readily let go. It iis not easy to switch overnight from yards to meters and from ounces to grams. And this is quite natural, because long familiarity with a unit gives us an idea of its magnitude and how it relates to the physical world.

        Nevertheless, the growing importance of SI (particularly in the electrical and mechanical fields) makes it necessary to know the essentials of this measurement system. Consequently, one must be able to convert from one system to another in a simple, unambiguous way.

        The SI possesses a number of remarkable features shared by no other system of units:

1. It is a decimal system.

2. It employs many units commonly used in industry and commerce; for example, volt, ampere, kilogram, and watt.

3. It is a coherent system that expresses with startling simplicity some of the most basic relationships in electricity, mechanics, and heat.

4. It can be used by the research scientist, the technician, the practicing engineer, and by the layman, thereby blending the theoritical and the practical worlds.

 Base and derived units of the SI

       The foundation of the International System of Units rests upon the seven base units listed in Table 1A.

                  

      From these base units we derive other units to express quantities such as area, power, force, magnetic flux, and so on. There is really no limit to the number of units we can derive, but some occur so frequently that they have been given special names. Thus, instead of saying that the unit of pressure is the newton per square meter, we use a less cumbersome name, the pascal. Some of the derived units that have special names are listed in Table 1B.

In this section, it will be explained some of these quantities;

Coulomb : is the quantity of electricity transported in 1 second by a current of 1 ampere.

Farad : is the capacitance of a capacitor between the plates of which there appears a difference of potential of 1 volt when it is charged by a quantity of electricity equal to 1 coulomb

Henry : is the inductance of a closed circuit which an electromotive force of 1 volt is produced when the electric current in the circuit varies uniformly at a rate of 1 ampere per second (Hence 1 henry = 1 volt second per ampere)

Hertz : is the frequency of a periodic phenomenon of which the period is 1 second.

Weber : is the magnetic flux that, linking a circuit of one turn, produces in it an electromotive force of 1 volt as it is reduced to zero at a uniform rate in 1 second (Hence 1 weber = 1 volt second)

Steradian : is the unit of measure of a solid angle with its vertex at the center of a sphere and enclosing an area of the spherical surface equal to that of a square with sides equal in length to the radius.

And also, in addition to them;

Resistivity(ohm meter) => Ωxm

Magnetic field strength (ampere per meter) => A / m