Almost all electric machines rotate about an axis, called the shaft of the machine. Because of the rotational nature of machinery, it is important to have a basic understanding of rotational motion. In this part, we will talk about a brief review of the concepts of distance, velocity, acceleration, Newton's law, and power as they apply to rotating machinery.
In general, a three-dimensional vector is required to completely describe the rotation of an object in space. However, machines normally turn on a fixed shaft, so its motion is restircted to one angular dimension. In the motion principle, direction of rotation can be described as either clockwise (CW) or counterclockwise (CCW). Counterclockwise angle of rotation is assumed to be positive, and a clockwise angle of rotation is assumed to be negative.
Angular Position θ
The angular position θ of an object is the angle at which it is oriented, measured from some arbitrary reference point. Usually, it is measured in radians or degrees.
Angular Velocity ω
Angular velocity or speed is the rate of change in angular position with respect to time. It is positive if the rotation is in a counterclockwise direction. Angular velocity is the rotational analog of the concept of velocity on a line.
ω = dθ /dt
If the units of angular position are radians, then angular velocity is measured in radian per second.
In dealing with ordinary electric machines, engineers often use units other than radians per second to describe shaft speed. Frequently, the speed is given in revolutions per second or revolutions per minute. Because speed is such an important quantity in the study of machines, it is customary to use different symbols for speed when it is expressed in different units. This symbols are used to describe angular velocity :
ωm = angular velocity expressed in radians per second
fm = angular velocity expressed in revolutions per second
nm = angular velocity expressed in revolutions per minute
nm = 60fm
fm = ωm / 2π
Angular Acceleration
Angular acceleration is the rate of change in angular velocity with respect to time. It is assumed positive if the angular velocity is increasing in an algebraic sense. Angular acceleration is defined by
α = d ω / dt
If the units of angular velocity are radians per second, then angular acceleration is measured in radians per second squared.
Torque τ
In linear motion, a force applied to an object causes its velocity to change. In the absence of a net force on the object, its velocity is constant. The greater the force applied to the object, the more rapidly its velocity changes.
There exists a similar concept for rotation. When an object is rotating, its angular velocity is constant unless torque is present on it. The greater the torque on the object, the more rapidly the angular velocity of the object changes.
The torque on an object is defined as the product of the force applied to the object and the smallest distance between the line of action of the force and the object's axis of rotation. If r is a vector pointing from the axis of rotation to the point of application of the force, and if F is the applied force, then the torque can be described as
τ=(Froce applied)(Perpendicular distance)
=(F)x(r)
=F.r.sinθ
where θ is the angle between the vector r and the vector F. The direction of the torque is clockwise if it would tend to cause a clockwise rotation and counterclockwise if it would tend to cause a counterclockwise rotation.
Newton's Law of Rotation
Simply, force applied on an object and its resulting acceleration.
F = net force applied on an object
m = mass of the object
a = resulting acceleration
F = m x a
A similar equation describes the relationship between the torque applied to an object and its resulting angular acceleration. This is called Newton's law of rotation ;
τ = is the net applied torque in newton-meters or pound-feet
I = serves the same purpose as an object's mass in linear motion. (moment of inertia)
α = is the resulting acceleration in radians per second squared
τ = I α
Work
For linear motion, work is defined as the application of a force through a distance. In equation form,
For rotational motion, work is the application of a torque through an angle. Here the equation for work is
and if the torque is constant
W = τθ
Power P
Power is the rate of doing work, or the increase in work per unit time. The equation for power is
P = dW / dt
it is usually measured in joules per second (watts), but also can be measured in foot-pounds per second or in horsepower.
By this definition, and assuming that force is constant and collinear with the direction of motion, power is given by
P = dW / dt
=d(F.r) / dt
= F ( dr / dt )
= F.v
Similarly, assuming constant torque, power in rotational motion is given by
P = dW / dt
=d(τθ) / dt
=τ(dθ / dt)
P =τω
This last power equation is very important in the study of electric machinery, because it can describe the mechanical power on the shaft of a motor or generator. This equation is the correct relationship among power, torque, and speed if power is measured in watts, torque in newton-meters, and speed in radians per second. If other units are used to measure any of the above quantities, then a constant must be introduced into the equation for unit conversion factors. It is still common in U.S. engineering practice to measure torque in pound-feet, speed in revolutions per minute, and power in either watts or horsepower. If the appropriate conversion factors are included in each term, then equation becomes
P (watts) = torque (Ib-ft) n (r / min) / 7.04
P (horsepower) = torque (Ib-ft) n (r / min) / 5252
where torque is measured in poun-feet and speed is measured in revolution per minute.

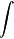
Hiç yorum yok:
Yorum Gönder